Economics
11.09.2022 • 7 min read
Subject Matter Expert
Learn what profit maximization is and why it is important. We’ll also go over the formula, how to calculate it, and look at examples.
In This Article
Imagine you are a small business owner. Maybe you’re running an Airbnb, a flower shop, or a small bakery. Aside from getting joy or satisfaction from the work you do, what is one thing that would likely motivate you?
As a business owner, you want to make as much money as you can. Economists refer to this as the profit motive. They assume that most companies in the economy operate with the goal of maximizing profits. To maximize profits, businesses need to identify a level of production—and sometimes a selling price—that will lead to a maximum profit. In this article, you’ll learn to model profit maximization the way economists do.
In economics, we assume that most businesses try to maximize profits. Profits are the difference between total revenue (the total amount of money a business earns from its customers) and total costs (the sum of all production costs of running the business).
The equation for profits is:
Where:
= profits
TR = total revenue
TC = total costs
Because profits are the difference between total revenue and total costs, profits are maximized where the difference between these two quantities is the greatest.
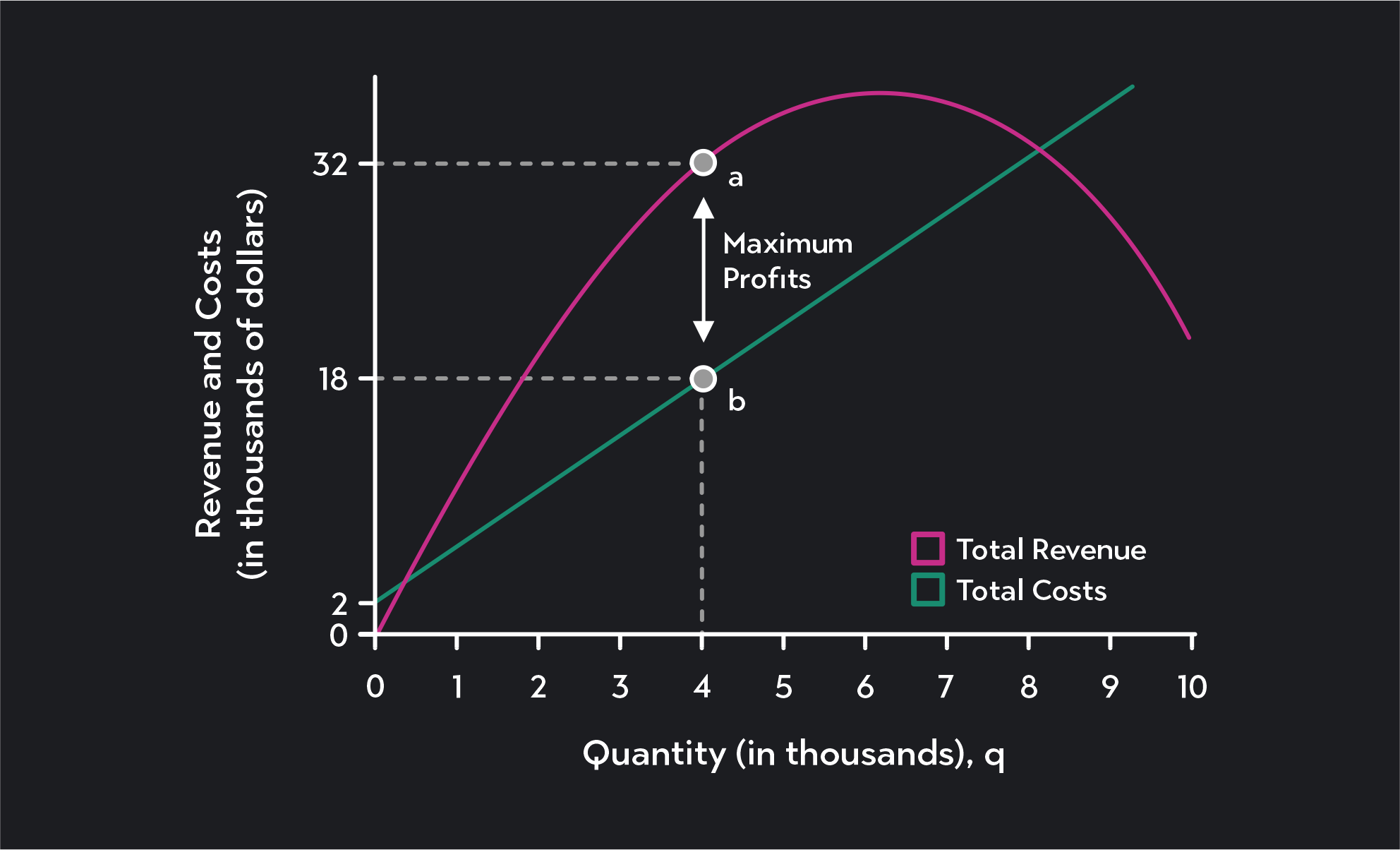
The graph below shows how total revenue and total costs vary as the quantity of output changes. For most firms, total revenue follows an upside-down, u-shaped pattern, and total costs increase with output. A firm’s profit-maximizing quantity, Q, is where the gap between total revenue and total costs is the greatest.
In this example, the profit-maximizing quantity is 4,000. At this quantity, the firm’s profits will be $14,000—the difference between $32,000 in revenue and $18,000 in costs. There is no other quantity on this graph where profits are higher.
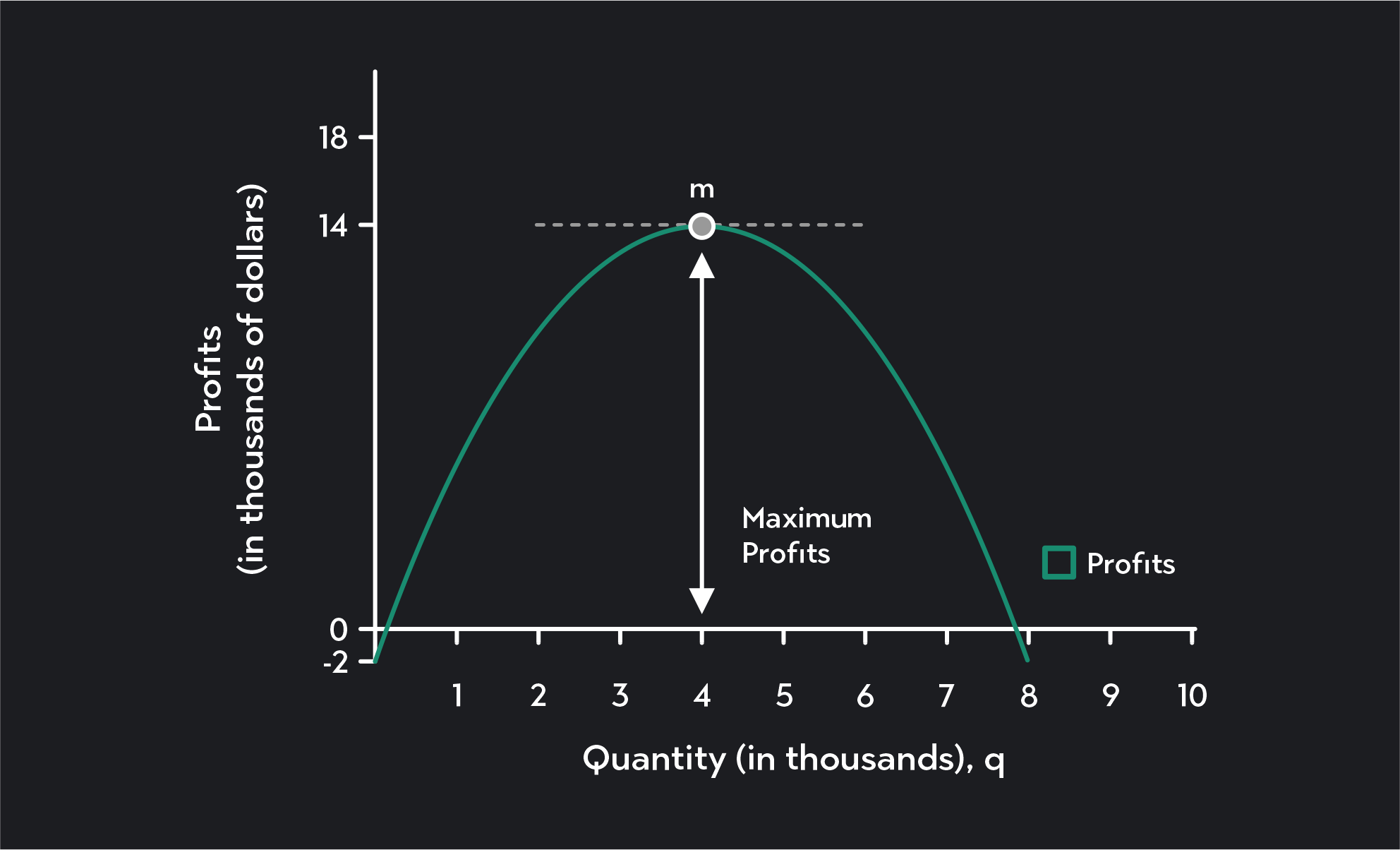
If we were to map out a corresponding graph of total profits, it would look like this. When the firm produces less than 4,000 units, it can increase profits by increasing the quantity it produces and sells. Profits are maximized at a quantity of 4,000 (as we already saw), beyond which point, the firm’s profit begins to fall.
You can also think about profit maximization at the margin. Thinking about something “at the margin” means thinking incrementally. You are assessing profits with every decision to produce one more or one less unit of output.
The marginal analysis of profit maximization involves comparing marginal revenue (MR) to marginal costs (MC) rather than comparing total revenue (TR) to total costs (TC).
Marginal revenue is the additional revenue gained from selling an additional (i.e., marginal) unit of your product. Marginal cost (MC) is the cost of producing the additional unit.
As a general rule, increasing output by one unit is always profitable if the marginal revenue gained from producing that unit is greater than the marginal cost of producing it. So long as MR > MC, producing the good will add to your profits. If the opposite is true—if marginal cost is greater than marginal revenue—producing the additional good reduces total profits.
As an example, imagine you produce bars of soap. Suppose you could sell an additional bar of soap for $5, but it would cost you $7 to produce it. Would you do it? No. You would lose $2. If instead, you could sell an additional bar of soap for $5, it would only cost you $2 to produce it. Selling the bar of soap would be profitable. It would provide you with $3 in profits.
The profit maximization rule takes the marginal analysis of profit maximization a step further. It states that businesses maximize profits by choosing a level of output, Q, where marginal revenue equals marginal costs.
The profit maximization rule holds for any type of business regardless of the type of market it operates in. It applies to firms that are monopolies and oligopolies, and it applies to firms in competitive markets.
Let’s look at an example of how the profit maximization rule works for a perfectly competitive firm.
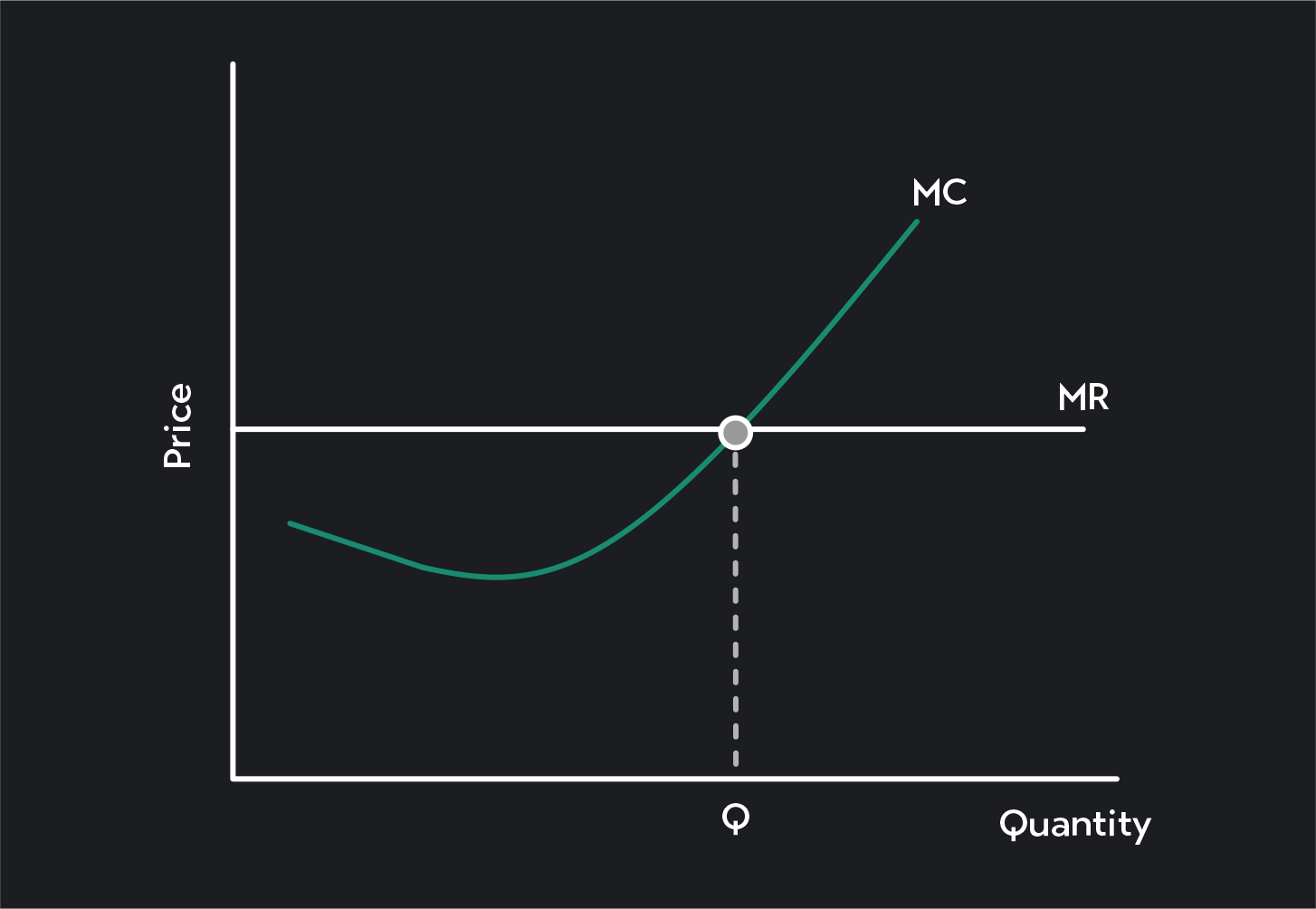
In a perfectly competitive market, firms are price takers, and each firm’s marginal revenue equals the market price.
For perfectly competitive firms:
This means that on a graph, the marginal revenue curve is a horizontal line that cuts across the graph at the market price.
Marginal costs, on the other hand, follow a pattern resembling a Nike swoosh. They decrease for some initial range of output but then start to increase. The initial decrease in marginal costs results from the firm’s fixed costs being spread over a larger quantity of output. The increase in marginal costs kicks in when the firm starts to experience diminishing marginal productivity.
The firm maximizes profits at quantity Q, where MR = MC. As we saw earlier, so long as marginal revenue exceeds marginal cost, the firm should continue to increase output incrementally. Following this logic, the firm continues increasing output until the quantity, Q. At this quantity, marginal revenue equals marginal cost, and the firm will maximize profits. Past this point, marginal costs will overtake marginal revenue, and the firm’s profits will start to diminish. The production of an extra unit of output beyond point Q will lower the firm’s profits.
Next, let’s look at an example of how the profit maximization rule applies to a monopoly. A monopoly is a firm that is the only seller in a market. As the sole seller in the market, a monopoly has the power and ability to set prices.
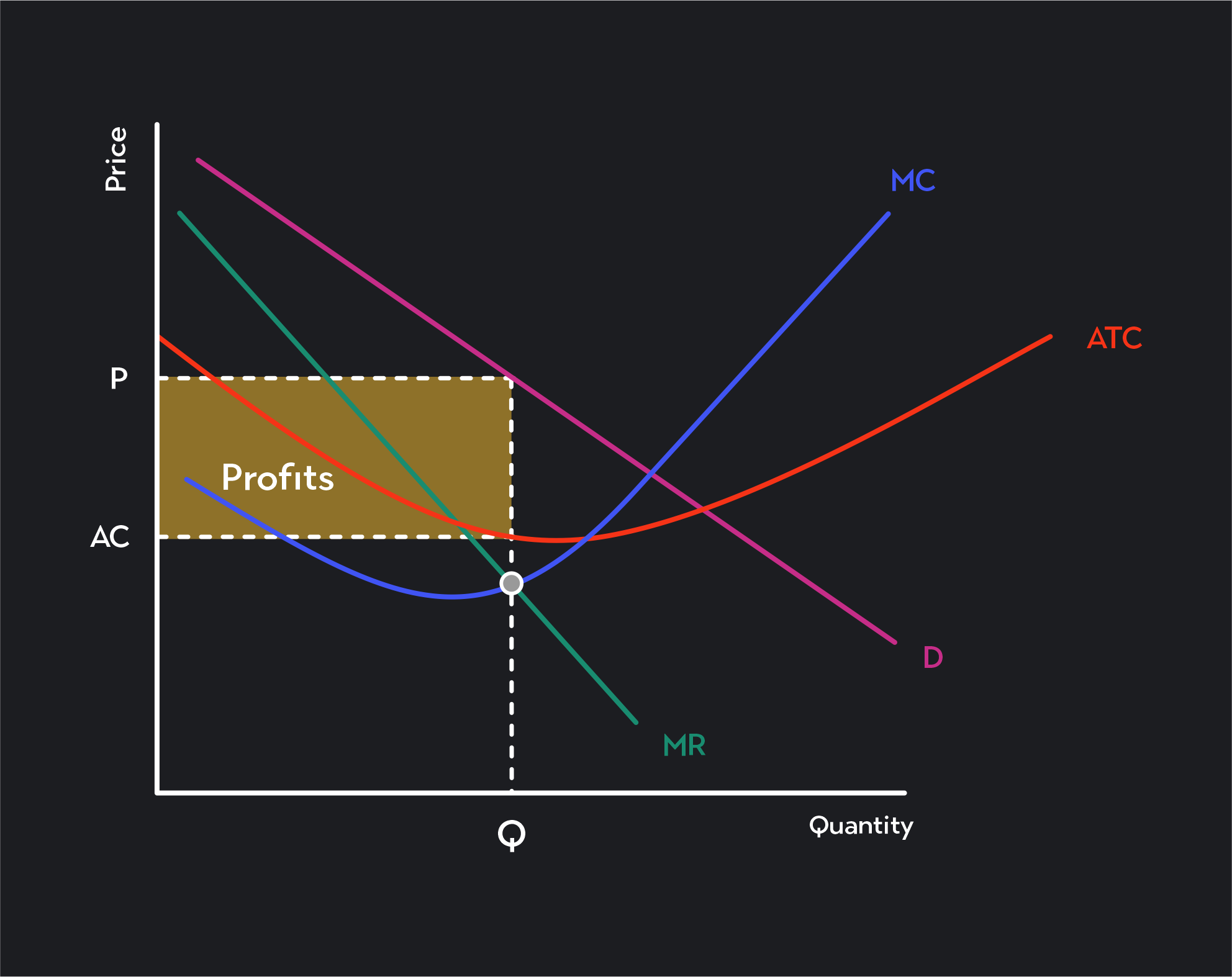
The monopolist’s marginal revenue curve is downward sloping as shown in the graph below. Marginal revenue decreases with output for a monopolist because of both the law of demand and the monopolist’s price setting power. A monopolist can choose what price to charge for their product, but they can only increase output if they are willing to lower their price per unit. If they want to charge a higher price, they have to lower their output.
The marginal cost curve for a monopolist is similar to the marginal cost curve for a competitive firm. They decrease for some initial amount of output and then begin to increase.
Following the profit-maximization rule, the monopolist chooses the output level where marginal revenue = marginal cost (MC = MR). In this example, that quantity is labeled Q*.
The monopolist can then find their profit-maximizing price by tracing the profit-maximizing quantity up to the demand curve and then across to the left to the price axis. In this case, the monopolist's profit-maximizing price is P*.
The rectangle shaded in yellow represents the monopolist’s profits. The base of the rectangle has the distance Q, and the height of the rectangle is equal to the profit maximizing price minus average total costs at the profit maximizing quantity.
The pursuit of profits is what encourages firms to innovate and produce goods that customers need and want. In this way, the profit motive is a big part of the engine that spurs economic growth. Modern economies would not be as prosperous without profit-seeking businesses.
Profit maximization also has some drawbacks. Firms that focus entirely on their own costs and revenues often produce and ignore negative externalities. Negative externalities are costs that individuals or firms impose on third parties. Pollution is a good example. A firm that produces goods while polluting the environment, does not consider the costs on society unless regulations curb or eliminate that behavior. In the absence of regulations, a firm that pollutes will produce a good or service that people want, but they will also produce a harm to society that is not part of their private cost-benefit analysis.
In the long run, firms can increase their profits by finding ways to increase revenue or lower costs.
Firms can increase their revenue by increasing the demand for their product. They do this by marketing their product and finding ways to differentiate their product from the goods of their competitors.
Firms can cut costs by identifying inefficiencies in their production process. They can also cut costs by developing or adopting technological innovations that allow them to produce their products with fewer or less costly inputs.
Outlier (from the co-founder of MasterClass) has brought together some of the world's best instructors, game designers, and filmmakers to create the future of online college.
Check out these related courses:
Economics
Learn what a perfectly competitive firm is, how they make output decisions, and how they determine the highest profit by comparing total revenue and total cost.
Subject Matter Expert
Economics
Here’s a simple explanation of how changes in demand and supply affect the equilibrium point with graphs and practice questions.
Subject Matter Expert
Economics
This article looks at marginal benefit vs marginal cost and the formula to calculate them. Read also about the different components, examples, and faqs.
Subject Matter Expert