If you could change one thing about college, what would it be?

Sample Standard Deviation- Phone with election map results- HighRes
Outlier Articles Home

Statistics

Sample Standard Deviation: What is It & How to Calculate It

12.15.2021 • 5 min read

Sarah Thomas

Subject Matter Expert

This article is a guide on sample standard deviation, including concepts, a step-by-step process to calculate it, and a list of examples.

In This Article

  1. What Is Standard Deviation?

  2. When to Use the Sample or Population Standard Deviation

  3. Sample Standard Deviation vs. Sample Variance

  4. How to Calculate a Sample Standard Deviation Step by Step

What Is Standard Deviation?

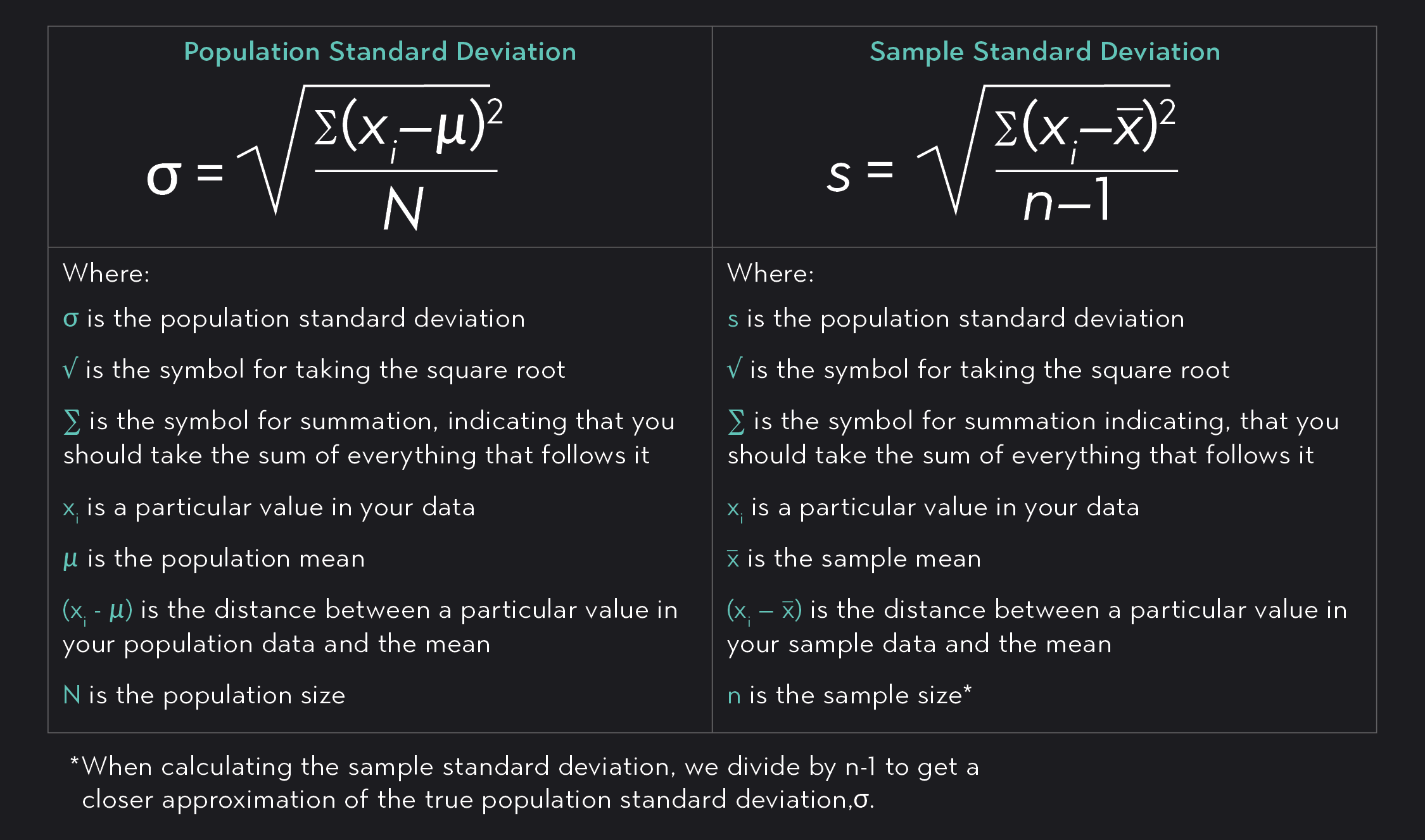
Standard deviation measures the spread of data relative to its mean. You can think of standard deviation as the average distance between a single data point and the mean. A large standard deviation shows that data is more widely dispersed about the mean, and a small standard deviation shows that data are tightly clustered around the mean. You can calculate standard deviation by taking the square root of the variance.

Sampling Standard Deviation Chart 1

When to Use the Sample or Population Standard Deviation

In statistics, a population refers to the entire set of objects or events being studied, and a sample is a subset of the population. As an example, imagine you are studying national elections in the United States. Your population of interest consists of every single eligible voter across the 50 states. Because you can’t collect data for the entire population, you draw random samples (subsets) of voters and make inferences about the population from these samples. Sometimes statisticians have data for an entire population. Most of the time, however, they have to work with samples.

When you are dealing with population data and want to calculate a standard deviation, use the population standard deviation formula given above. A population standard deviation is denoted by the lowercase Greek letter sigma, 𝞂.

When you are dealing with sample data and want to calculate a standard deviation, use the sample standard deviation formula given above. A sample standard deviation is denoted by the lowercase letter s.

Sample Standard Deviation vs. Sample Variance

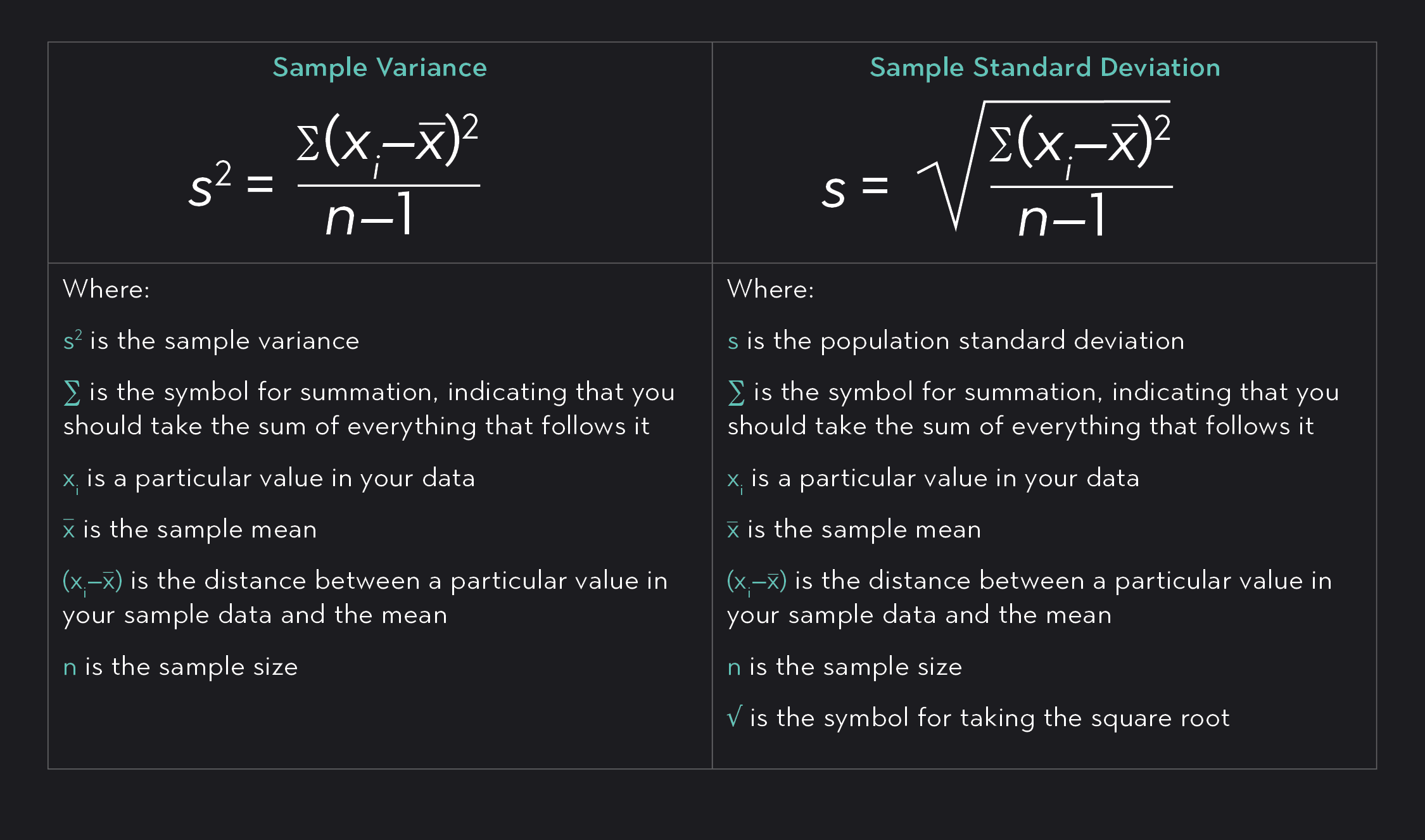
We calculate standard deviation as the square root of the variance. This may cause you to wonder: why do we use standard deviation when we already have variance? Great question!

To answer this question, first notice that in both the equation for variance and the equation for standard deviation, you take the squared deviation (the squared distances) between each data point and the sample mean (xi−xˉ)2(x_i-\bar{x})^2. You do this so that the negative distances between the mean and the data points below the mean do not cancel out the positive distances between the mean and data points above it.

By squaring deviations, we convert each deviation into a positive number, and from there, you can measure average dispersion. There is a downside to doing this, though. By squaring the deviations, you square the units of the data as well. Imagine your data is measured in ounces. By taking squared deviations, you are now measuring the dispersion in your data in terms of ounces squared — a unit of measurement that is difficult to interpret.

Standard deviation is intended to be a standardized measure of dispersion. By taking the square root of the variance, we convert the statistic back into the same units as the data. Ounces, not ounces squared!

Sampling Standard Deviation Chart 2

How to Calculate a Sample Standard Deviation Step by Step

Say you have the following sample data for temperatures measured in degrees Fahrenheit. For simplicity, this sample just has four observations (n=4).

Sample Data
{51 °F, 58 °F, 61 °F, 62 °F}

Using the formula for sample standard deviation, let’s go through a step-by-step example of how to find the standard deviation for this sample.

s=∑(xi−xˉ)2n−1s = \sqrt{\frac{\sum_{}^{}(x_i-\bar{x})^2}{n-1}}

STEP 1

Calculate the sample mean x̅.

xˉ=51+58+61+624=58°F\bar{x}=\frac{51+58+61+62}{4} = 58 \degree F

STEP 2

Calculate the deviation between each data point and the sample mean (xi−xˉ)(x_i-\bar{x}).

51−58=−751-58 = - 7
58−58=058-58 = 0
61−58=3°F61-58 = 3°F
62−58=4°F62-58 = 4°F

Notice that there are both positive and negative deviations.

STEP 3

Square the deviations, (xi−xˉ)2(x_i-\bar{x})^2.

(−7)2=49\left(-7\right)^2=49
02=0 0^2 = 0
32=93^2 = 9
42=164^2 =16

By squaring the deviations, we turn all of the deviations positive.

STEP 4

Sum the squared distances, ∑(xi−xˉ)2\sum(x_i-\bar{x})^2.

49+0+9+16=7449+0+9+16 = 74

STEP 5

Divide the sum of the squares by the number of data points minus one, ∑(xi−xˉ)2(n−1)\dfrac{\sum(x_i-\bar{x})^2}{(n-1)}.

Notice that this is the variance, s2s^2, and it is measured in degrees Fahrenheit squared!

STEP 6

Take the square root of the variance.

s=∑(xi−xˉ)2n−1=24.66ˉ=4.97s = \sqrt{\frac{\sum_{}^{}(x_i-\bar{x})^2}{n-1}} = \sqrt{24.6\bar{6}} = 4.97

And there is your sample standard deviation. 4.97 °F.

Explore Outlier's Award-Winning For-Credit Courses

Outlier (from the co-founder of MasterClass) has brought together some of the world's best instructors, game designers, and filmmakers to create the future of online college.

Check out these related courses:

Intro to Statistics

Intro to Statistics

How data describes our world.

Explore course
Intro to Microeconomics

Intro to Microeconomics

Why small choices have big impact.

Explore course
Intro to Macroeconomics

Intro to Macroeconomics

How money moves our world.

Explore course
Intro to Psychology

Intro to Psychology

The science of the mind.

Explore course

Share

Related Articles