A Guide to Price Elasticity of Demand

In This Article

1. What is Price Elasticity of Demand?

2. Calculating Price Elasticity

3. Zones of Elasticity

4. What Makes a Good Elastic or Inelastic?

5. Other Types of Elasticity

What is Price Elasticity of Demand?

Price elasticity of demand $E_D$ measures the change in the quantity demanded of a good relative to a change in its price.

We know from the Law of Demand that there is an inverse relationship between price and quantity demanded for most goods. As the price of a good increases, demand falls; as the price of a good decreases, demand rises. Price elasticity of demand tells us how big or small the resulting percentage change in the quantity demanded is relative to the percentage change in price.

For some goods, the percentage change in the quantity demanded is large relative to the price change. Such goods have a price elasticity of demand that is greater than 1. They are considered elastic.

For other goods, the percentage change in demand is small relative to the price change. These goods have an elasticity that is less than 1. They are considered inelastic.

Calculating Price Elasticity

To calculate the price elasticity of demand, we need to find the ratio of the percentage change in the quantity demanded to the percentage change in price. (Percentage change can be calculated by subtracting the original value from the new value, and then dividing this by the original value.) This ratio uses the absolute value of each percentage change because we only compare the magnitude of the changes and not the direction.

$\text{Price Elasticity of Demand ($E_D$)} = \dfrac{|\text{\% Change in the Quantity Demanded}|}{|\text{\% Change in the Price}|}$

Notice that the elasticity will be greater than 1 when the numerator is greater than the denominator. In other words, elasticity will be greater than 1 when the percentage change in quantity is greater than the percentage change in price. Likewise, the elasticity will be less than 1 if the percentage change in quantity is less than the percentage change in price. This is how we determine whether a good is elastic (E_D>1) or inelastic (E_D<1).

Let’s work through an example.

Say a health food chain increases the price of their smoothies from $10 to $12 and as a result, the number of smoothies demanded (i.e., the quantity demanded) falls from 5,000 to 3,500. You can see these changes represented as the change from Point A to Point B on the demand curve below.

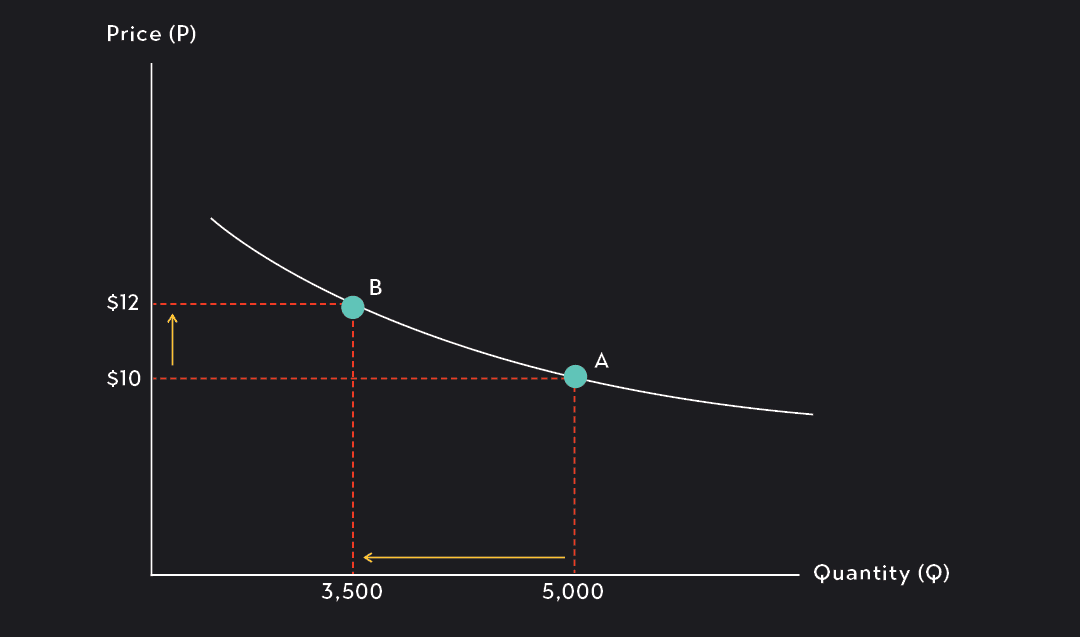
We can calculate the price elasticity of demand in three easy steps.

Step 1. Find the percentage change in price First, we find the percentage change in price, the denominator in our price elasticity of demand equation. To do this, we subtract the original price from the new price and divide the difference by the original price. Therefore, an increase in price from $10 to $12 is equal to 0.20 or a 20% increase.

$\%Change\;in\;Price=\frac{New\;Price\;-\;Original\;Price}{Original\;Price}=\frac{\$12-\$10}{\$10}=0.2=20\%$

Step 2. Find the percentage change in quantity

Next, we find the percentage change in the quantity demanded using the exact same method we used for the percentage change in price. In this case, a decrease from 5,000 smoothies to 3,500 smoothies equals 0.3, or a 30% decrease.

$\%Change\;in\;Quantity\;Demanded=\frac{New\;Quantity\;-\;Original\;Quantity}{Original\;Quantity}=\frac{3500-5000}{5000}=-0.3=-30\%$

Step 3. Find the price elasticity of demand using the absolute values of the changes found in Steps 1 and 2

To find the price elasticity of demand, we take the absolute value of the percentage changes we found in Steps 1 and 2. Then, we divide the percentage change in quantity by the percentage change in price.

$E_D\;for\;Smoothies\;at\;a\;Health\;Foods\;Chain=\frac{0.3}{0.2}=1.5$

In this example, we find an elasticity of 1.5, which is greater than 1. We know from earlier that this indicates that the demand for the smoothies is elastic: the percentage change in the quantity demanded is greater than the percentage change in price. In this case, a 20% increase in price resulted in a 30% decrease in demand. The percentage change in demand was 1.5 times greater than the change in price. Hence, elasticity($E_D$) = 1.5.

Zones of Elasticity

We’ve already established that goods with an elasticity greater than 1 are elastic and that goods with an elasticity less than 1 are inelastic. Now, we can further designate elasticity into five categories or five zones. These are shown in the charts below.

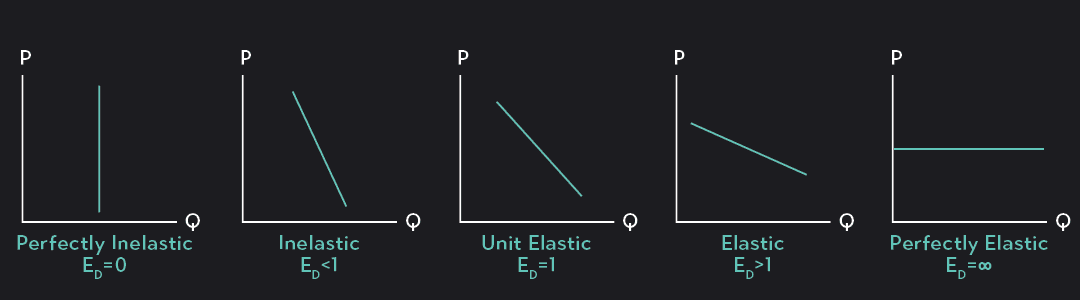
Perfectly Inelastic Goods ($E_D$=0): When the price elasticity of demand is equal to zero, the demand is perfectly inelastic. This means that price changes will have no effect on the quantity demanded. The demand curve for such goods is a vertical line indicating that the quantity demanded will remain unchanged no matter what price is charged. Perfectly inelastic demand is rare, but it can occur when a good is an absolute necessity. Demand for a life-saving drug, for example, could be perfectly inelastic: people who need it may be willing to pay any price to get it.

Inelastic Goods (E_D < 1): When demand for a good is inelastic, the demand is relatively resistant to changes in price, and thus, the percentage change in quantity demanded will be less than the percentage change in price. Inelastic goods have an elasticity of less than 1 and have steep demand curves (but not vertical).

Unit Elastic Goods ($E_D$ = 1): A unit elastic good has a price elasticity of demand equal to 1. In such cases, the percentage change in quantity demanded is equal to the percentage change in price. So if the price increases by 15%, demand will fall by 15%, or if the price decreases by 5%, demand will rise by 5%.

Elastic Goods ($E_D$ > 1): When demand for a good is elastic, the demand is relatively sensitive to changes in the price, as we saw in our smoothie example. Any change in the product’s price will lead to a larger percentage change in the quantity demanded. Elastic goods have an elasticity of less than 1 and have flatter (but not quite horizontal) demand curves.

Perfectly Elastic Goods ($E_D$ = ∞): Finally, perfectly elastic goods are the extreme opposite of perfectly inelastic goods. In this case, even a slight change in price will cause demand to drop to zero. This can happen when a good has plenty of close substitutes. For example, a can of non-organic black beans costs about $1. If a single company tries to raise the price of their canned black beans, consumers can quickly abandon the company’s product and buy beans from a different provider. The demand curve for perfectly elastic goods is horizontal, indicating that any deviation from the existing price will cause demand to drop to zero.

The price elasticity of demand is not equal to the slope of the demand curve.

Because the elasticity of demand is related to the slope of the demand curve, it’s easy to confuse the two, but be careful. The slope of the demand curve and the price elasticity of demand are not the same things. 

The slope of the demand curve is approximated by the change in price divided by the change in quantity. The price elasticity of demand is calculated as the percentage change in quantity divided by the percentage change in price!

$Slope\;of\;the\;Demand\;Curve=\frac{Change\;in\;Price}{Change\;in\;Quantity}=\frac{\Delta P}{\Delta Q}$

$Price\;Elasticity\;of\;Demand=\frac{Percentage\;Change\;in\;Quantity}{Percentage\;Change\;in\;Price}=\frac{\%\Delta Q}{\%\Delta P}$

What Makes a Good Elastic or Inelastic?

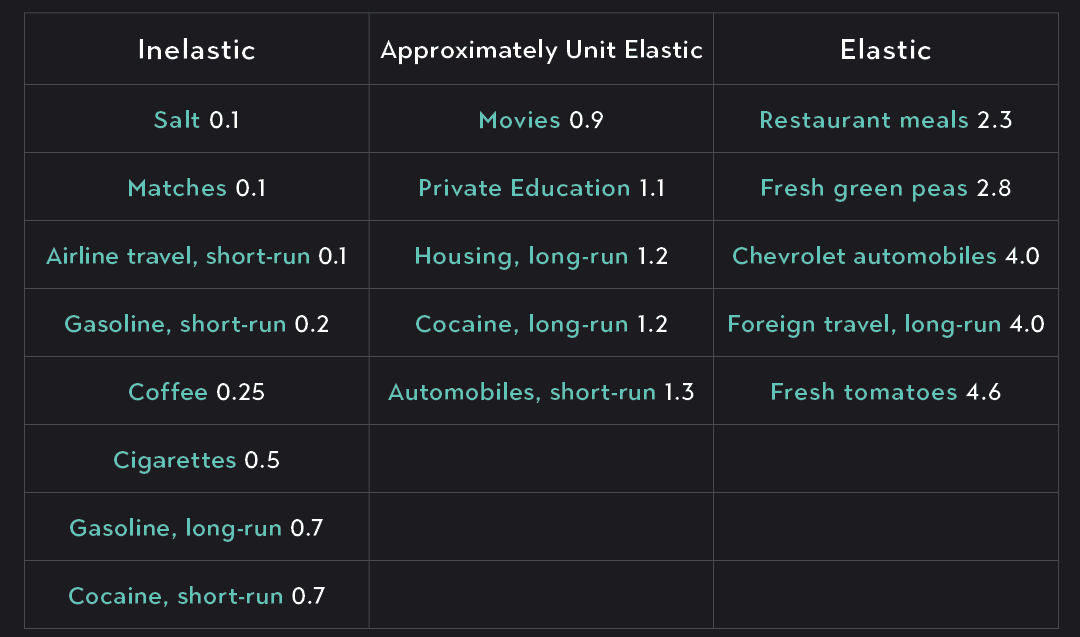
What makes the demand for a good elastic or inelastic? The chart below lists some common goods with their estimated price elasticities of demand. We can use these to help us understand factors that influence elasticity.

The main factors that influence the elasticity of demand are the following:

The availability of close substitutes

When a good has plenty of close substitutes, consumers can easily reduce their demand for the good and switch over to the substitutes (demand will be elastic). We saw this with the example of canned black beans earlier. This is also why restaurant meals are elastic. Consumers can switch to cheaper restaurants or eat at home more frequently if restaurants raise their prices.

On the other hand, when there are few or no available substitutes, consumers have a harder time adjusting their consumption in response to price changes (demand will be inelastic). This explains why goods such as coffee or cigarettes are inelastic. For people who rely on these goods, there are no close substitutes.

Whether the good is a necessity or a luxury

When the price of a luxury good increases, consumers can easily decide to reduce consumption or forgo buying the good altogether, and so demand will be elastic. This explains the elasticity of things such as foreign travel and fancy automobiles. These goods are not necessary, so they can more easily be given up.

On the other hand, if a good is a necessary item, consumers have a harder time adjusting their consumption in response to a price change, and so demand is inelastic. Gasoline, for example, is inelastic because people rely on it to get to work every day.

The portion of income spent on the good

When a good makes up a significant portion of a consumer’s overall budget or income, the consumer is likely to be more sensitive to changes in price (i.e., demand will be elastic). This is true for goods and services such as vacation homes, additional automobiles, or cosmetic surgeries.

On the other hand, when a good makes up an insignificant amount of a consumer’s overall budget or income, the consumer may be more willing to continue purchasing the product despite changes in the price (i.e., demand will be inelastic). This is why salt and matches fall into the inelastic category. Even if a salt grinder doubled in price (a 100% increase), you may only be paying a dollar or two extra out of your entire income.

The time consumers have to adjust to price changes

In many instances, goods are more elastic in the long term, because consumers have more time to adjust their behavior to changes in price. For example, someone who smokes cigarettes or is addicted to drugs may change their behavior and reduce consumption over longer periods. Even for necessary goods such as gasoline, people can adjust their behavior by organizing carpools or finding alternative modes of transportation. Still, changing habits and finding alternatives takes time.

On the other hand, goods tend to be more inelastic in the short term. If the price of gasoline goes up overnight, you still need to get to work the next morning. If you have a strong enough need or if you are addicted to a good, changing your habits in an instant can be difficult.

Other Types of Elasticity

In this brief, you learned about the price elasticity of demand, but you should know that there are other types of elasticity as well. These include:

Cross-Price Elasticity of Demand

This measures the change in the quantity demanded of one good relative to a price change in another good. This comes in handy when you are trying to estimate the relationship between demand for one good and the price of another related good, such as a compliment or a substitute.

Income Elasticity of Demand

This measures the change in demand for a good relative to a change in consumer income.

Price Elasticity of Supply

This measures the change in the quantity of a good that a producer is willing to supply relative to changes in the price they can charge.

The calculations for these different types of elasticities are slightly different from one another. However, the basic intuition behind all of them is the same. In each case, the elasticity measures the responsiveness (or the resulting change) in one factor—typically the quantity demanded or supplied of a good—relative to some other factor such as price or income.

Explore Outlier's Award-Winning For-Credit Courses

Outlier (from the co-founder of MasterClass) has brought together some of the world's best instructors, game designers, and filmmakers to create the future of online college.

Check out these related courses:

Intro to Microeconomics

Why small choices have big impact.

Explore course

Intro to Microeconomics

Why small choices have big impact.

Explore course

Intro to Macroeconomics

How money moves our world.

Explore course

Intro to Macroeconomics

How money moves our world.

Explore course

Intro to Statistics

How data describes our world.

Explore course

Intro to Statistics

How data describes our world.

Explore course

College Algebra

Math rules everything around us.

Explore course

College Algebra

Math rules everything around us.

Explore course